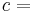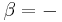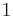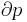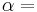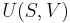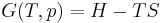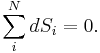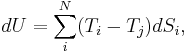Zeroth law of thermodynamics

Thermodynamics
Laws
Zeroth · First · Second · Third

The zeroth law of thermodynamics is a generalization principle of thermal equilibrium among bodies, or thermodynamic systems, in contact.

The zeroth law states that if two systems are in thermal equilibrium with a third system, they are also in thermal equilibrium with each other.

Systems are said to be in thermal equilibrium if they do not actually exchange heat, and, if they are not already connected by a conductor of heat or pathway for exchange of thermal radiation, would not do so if they were so connected. The law implies that thermal equilibrium between systems is a transitive relation, which affords the definition of an empirical physical parameter, called temperature. The temperatures are equal for all systems in thermal equilibrium. The law permits the construction of a thermometer to measure this property.[1]

Contents

Zeroth law as equivalence relation

A system is said to be in thermal equilibrium when it experiences no net change in thermal energy. If A, B, and C are distinct thermodynamic systems, the zeroth law of thermodynamics can be expressed as:[2]

"If A and C are each in thermal equilibrium with B, A is also in equilibrium with C."

This statement asserts that thermal equilibrium is a Euclidean relation between thermodynamic systems. If we also grant that all thermodynamic systems are in thermal equilibrium with themselves, then thermal equilibrium is also a reflexive relation. Relations that are both reflexive and Euclidean are equivalence relations. One consequence of this reasoning is that thermal equilibrium is a transitive relationship: If A is in thermal equilibrium with B and B is in thermal equilibrium with C, then A is in thermal equilibrium with C. Another consequence is that the equilibrium relationship is symmetric: If A is in thermal equilibrium with B, then B is in thermal equilibrium with A. Thus we may say that two systems are in thermal equilibrium with each other, or that they are in mutual equilibrium. Implicitly assuming both reflexivity and symmetry, the zeroth law is therefore often expressed as[3]:

"If two systems are in thermal equilibrium with a third system, then they are in thermal equilibrium with each other"

Again, implicitly assuming both reflexivity and symmetry, the zeroth law is occasionally expressed as the transitive relationship [4]

"If A is in thermal equilibrium with B and if B is in thermal equilibrium with C, then A is in thermal equilibrium with C."

Thermal equilibrium between many systems

Many systems are said to be in equilibrium if the small, random exchanges (due to Brownian motion or photon emissions, for example) between them do not lead to a net change in the total energy summed over all systems. A simple example illustrates why the zeroth law is necessary to complete the equilibrium description.

Consider N systems in adiabatic isolation from the rest of the universe, i.e. no heat exchange is possible outside of these N systems, all of which have a constant volume and composition, and which can only exchange heat with one another.

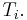The combined First and Second Laws relate the fluctuations in total energy, d U, to the temperature of the ith system, \ T_i. and the entropy fluctuation in the ith system, 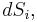\ d S_i, as follows:

d U=\sum_i^NT_id S_i.

The adiabatic isolation of the system from the remaining universe requires that the total sum of the entropy fluctuations vanishes, or:

\sum_i^Nd S_i=0.

That is, entropy can only be exchanged between the N systems. This constraint can be used to rearrange the expression for the total energy fluctuation and obtain:

d U=\sum_{i}^N(T_i-T_j)d S_i,

where \ T_j is the temperature of any system j we may choose to single out among the N systems. Finally, equilibrium requires the total fluctuation in energy to vanish, in which case:

\sum_{i}^N(T_i-T_j)d S_i=0,

which can be thought of as the vanishing of the product of an antisymmetric matrix 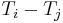\ T_i-T_j and a vector of entropy fluctuations 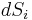\ d S_i. In order for a non-trivial solution to exist,

d S_i\ne 0.

That is, the determinant of the matrix formed by 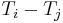\ T_i-T_j must vanish for all choices of N. However, according to Jacobi's theorem, the determinant of a NxN antisymmetric matrix is always zero if N is odd, although for N even we find that all of the entries must vanish, 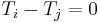\ T_i-T_j=0, in order to obtain a vanishing determinant. Hence \ T_i=T_j at equilibrium. This non-intuitive result means that an odd number of systems are always in equilibrium regardless of their temperatures and entropy fluctuations, while equality of temperatures is only required between an even number of systems to achieve equilibrium in the presence of entropy fluctuations.

The zeroth law solves this odd vs. even paradox, because it can readily be used to reduce an odd-numbered system to an even number by considering any three of the N systems and eliminating one by application of its principle, and hence reduce the problem to even N which subsequently leads to the same equilibrium condition that we expect in every case, i.e., \ T_i=T_j. The same result applies to fluctuations in any extensive quantity, such as volume (yielding the equal pressure condition), or fluctuations in mass (leading to equality of chemical potentials). Hence the zeroth law has implications for a great deal more than temperature alone. In general, we see that the zeroth law breaks a certain kind of asymmetry present in the First and Second Laws.

Foundation of temperature

The zeroth law establishes thermal equilibrium as an equivalence relationship. An equivalence relationship on a set (such as the set of thermally equilibrated systems) divides that set into a collection of distinct subsets ("disjoint subsets") where any member of the set is a member of one and only one such subset. In the case of the zeroth law, these subsets consist of systems which are in mutual equilibrium. This partitioning allows any member of the subset to be uniquely "tagged" with a label identifying the subset to which it belongs. Although the labeling may be quite arbitrary,[5] temperature is just such a labeling process which uses the real number system for tagging. The zeroth law justifies the use of suitable thermodynamic systems as thermometers to provide such a labeling, which yield any number of possible empirical temperature scales, and justifies the use of the second law of thermodynamics to provide an absolute, or thermodynamic temperature scale. Such temperature scales bring additional continuity and ordering (i.e., "hot" and "cold") properties to the concept of temperature.[3]

In the space of thermodynamic parameters, zones of constant temperature form a surface, that provides a natural order of nearby surfaces. One may therefore construct a global temperature function that provides a continuous ordering of states. The dimensionality of a surface of constant temperature is one less than the number of thermodynamic parameters, thus, for an ideal gas described with three thermodynamic parameters P, V and n, it is a two-dimensional surface.

For example, if two systems of ideal gases are in equilibrium, then P1V1/N1 = P2V2/N2 where Pi is the pressure in the ith system, Vi is the volume, and Ni is the amount (in moles, or simply the number of atoms) of gas.

The surface PV/N = const defines surfaces of equal thermodynamic temperature, and one may label defining T so that PV/N = RT, where R is some constant. These systems can now be used as a thermometer to calibrate other systems. Such systems are known as "ideal gas thermometers".

History

Temperature has long been known as a quality of heat, for example, to Galileo and to Newton. Carnot took it as a presupposition for his work. Thermometers may be described as empirical or absolute. Absolute thermometers are calibrated numerically by the thermodynamic absolute temperature scale. It was not until the middle of the nineteenth century that absolute thermodynamic temperature was recognized, long after the recognition of empirical thermometry.

Empirical thermometry recognizes hotness as a fundamental character of temperature and thermometers.[6][7][8] Empirical thermometers are not in general necessarily in exact agreement with each other or with absolute thermometers as to their numerical scale readings, but to qualify as thermometers at all they must agree with absolute thermometers and with each other in the following way: given any two bodies isolated in their separate respective thermodynamic equilibrium states, all thermometers agree as to which of the two has the higher temperature, or that the two have equal temperatures. For any two empirical thermometers, this does not require that the relation between their numerical scale readings be linear, but it does require that relation to be strictly monotonic.[9]

Truesdell reports that Rankine wrote in 1853:

Definition of equal temperatures.
Two portions of matter are said to have equal temperatures, when neither tends to communicate heat to the other.[10]

Discussing the concept of temperature, James Clerk Maxwell in 1872 wrote: "If when two bodies are placed in thermal communication, one of the two bodies loses heat, and the other gains heat, that body which gives out heat is said to have a higher temperature than that which receives heat from it." He drew the corollary "If when two bodies are placed in thermal communication neither of them loses or gains heat, the two bodies are said to have equal temperatures or the same temperature. The two bodies are then said to be in thermal equilibrium."

Further, Maxwell stated, as the "Law of equal temperatures" the following triviality: "Bodies whose temperatures are equal to that of the same body have themselves equal temperatures".[11] Maxwell then offered an argument that this statement was "not a truism". Later in the same text, Maxwell wrote: "Hence the result of the conduction and radiation of heat from one part of a system to another is to diminish the entropy of the system, or the energy, available as work, which can be obtained from the system." This statement was surrounded in Maxwell's text by several others like it that show that it was no slip of the pen. In the same textbook, Maxwell wrote[12] that he was following Tait in re-defining the word entropy that had been introduced by Clausius. In contrast with what Maxwell wrote then, Tait had changed his mind by 1884 when in his text he accepted Clausius's original definition of entropy.[13]

Subsequent writers made statements like Maxwell's. Tait in 1884 wrote "if A is at the same temperature as B and also at the same temperature as C — no transfer of heat takes place between B and C, whatever be these bodies."[14] A similar statement was made by Max Planck in 1897, not labeled as a law but as an important proposition: "If a body, A, be in thermal equilibrium with two other bodies, B and C, then B and C are in thermal equilibrium with one another."[15] Planck repeated this important proposition in the seventh edition of his treatise in 1922.

The title "zeroth law of thermodynamics" began to appear in textbooks to refer to statements of this kind, though now stripped of their explicit reference to heat; their implicit dependence on the notion of heat could not be removed because they rely on the concept of thermal equilibrium which in turn relies on the concept of transfer of heat by conduction or radiation, the presence or absence of which must be empirically recognizable in order to make the concept of thermal equilibrium empirically recognizable. An early example is in the textbook of statistical thermodynamics of Fowler and Guggenheim (1939/1965).[16] Their focus of interest in that book was homogeneous systems (page 1), which they termed 'assemblies'. They dealt with assemblies that were either completely homogeneous or that could be divided into homogeneous parts, called phases (page 58). For their macroscopic thermodynamic account of phenomena, they started by accepting, on empirical physical grounds, the presupposed notions of thermal insulation, thermal contact, and thermal equilibrium (page 56). They emphasized that these notions "can be defined without any reference to temperature" (page 56). Moreover, they gave at this stage of their development of their theory no hint of the notion of heat transfer. On page 56, they wrote:

...we introduce the postulate: If two assemblies are each in thermal equilibrium with a third assembly, they are in thermal equilibrium with each other.

They then proposed that "it may be shown to follow that the condition for thermal equilibrium between several assemblies is the equality of a certain single-valued function of the thermodynamics states of the assemblies, which may be called the temperature t, any one of the assemblies being used as a "thermometer" reading the temperature t on a suitable scale. This postulate of the "Existence of temperature" could with advantage be known as the zeroth law of thermodynamics" (page 56). They did not there state any reason why such a function should have values in a scale consisting of a continuous succession of numbers or that it should have anything to do with heat. Their thinking was apparently conditioned by their asserted belief that "The most logically satisfactory formulation [of homogeneous system macroscopic thermodynamics] is undoubtedly that of Carathéodory" (page 56). Though they thus apparently professed concern for logicality, they had no apparent compunction about immediately assuming, without apparent justification, that their postulated "temperatures" should exist on a numerical scale, provided for example by "the measured volume of a constant quantity of any chosen substance at constant pressure" (page 56). No worries about pesky but relevant physical realities such as the anomalous behaviour of water[17] that concerned the nineteenth century thermodynamicists, because around 4 C it does not provide a valid empirical temperature. No mention that it would therefore be safer to refer to a permanent gas as a thermometric material. The approach of Fowler and Guggenheim is labeled "mechanical" by Bailyn,[18] who contrasts it with the "thermodynamic" approach of Planck and the founders, who fully recognized the notion of heat transfer as an essential and fundamental presupposition to thermodynamics, without actually labelling it as a numbered law of thermodynamics. Since the time of Fowler and Guggenheim, who believed that the 1909 axiomatic formulation of Carathėodory was the most logically satisfactory, other influential axiomatic formulations of thermodynamics have appeared, some of which do not refer to a zeroth law of thermodynamics.[19][20]

Sommerfeld in 1951 gave the title the "Zeroth Law" to the statement "Equality of temperature is a condition for thermal equilibrium between two systems or between two parts of a single system"; he wrote that this title followed the suggestion of Fowler, made when he was giving an account of a certain book.[21] Sommerfeld's statement took the existence of temperature for granted, and used it to specify one of the characteristics of thermodynamic equilibrium. This is converse to many statements that are labeled as the zeroth law, which take thermal equilibrium for granted and use it to contribute to the concept of temperature. We may guess that Fowler had made his suggestion because the notion of temperature is in effect a presupposition of thermodynamics that earlier physicists had not felt needed explicit statement as a law of thermodynamics, and because the mood of his time, pursuing a "mechanical" axiomatic approach, wanted such an explicit statement.

Guggenheim in 1966 wrote "If two systems are both in thermal equilibrium with a third system then they are in thermal equilibrium with each other" as the zeroth law of thermodynamics, and followed it with the comment "In other words, systems in thermal equilibrium are said to have the same temperature."[22] This ordinary language statement, although less precise than the statements of Planck and Tait mentioned above, conveys much of the essence of the zeroth law.

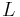The statement of the zeroth law of thermodynamics by Serrin in 1977, though rather mathematically abstract, is more informative for empirical thermometry: "Zeroth Law - There exists a topological line M which serves as a coordinate manifold of material behaviour. The points L of the manifold M are called 'hotness levels', and M is called the 'universal hotness manifold'."[23] To this information there needs to be added a sense of greater hotness; this sense can be had, independently of calorimetry, of thermodynamics, and of properties of particular materials, from Wien's displacement law of thermal radiation: the temperature of a bath of thermal radiation is proportional, by a universal constant, to the frequency of the maximum of its frequency spectrum; this frequency is always positive, but can have values that tend to zero.

References

  1. ^ Reif, F. (1965). "Chapter 3.5: Temperature". Fundamentals of Statistical and Thermal Physics. New York: McGraw-Hill. pp. 102ff. LCCN 63-22730. 
  2. ^ Chris Vuille; Serway, Raymond A.; Faughn, Jerry S. (2009). College physics. Belmont, CA: Brooks/Cole, Cengage Learning. pp. 355. ISBN 0-495-38693-6. 
  3. ^ a b H.A. Buchdahl (1966). The Concepts of Classical Thermodynamics. Cambridge University Press. p. 73. 
  4. ^ D. Kondepudi (2008). Introduction to Modern Thermodynamics. Wiley. p. 7. http://www.amazon.com/Introduction-Modern-Thermodynamics-Dilip-Kondepudi. 
  5. ^ J. S. Dugdale (1996, 1998). Entropy and its Physical Interpretation. Tayler & Francis. ISBN 9-7484-0569-0. 
  6. ^ Mach, E. (1900). Die Principien der Wärmelehre. Historisch-kritisch entwickelt, Johann Ambrosius Barth, Leipzig, section 22, pages 56-57.
  7. ^ Truesdell, C.A. (1980). The Tragicomical History of Thermodynamics, 1822-1854, Springer, New York, ISBN 0-387-90403-4.
  8. ^ Serrin, J. (1986). Chapter 1, 'An Outline of Thermodynamical Structure', pages 3-32, especially page 6, in New Perspectives in Thermodynamics, edited by J. Serrin, Springer, Berlin, ISBN 3-540-15931-2.
  9. ^ Thomsen, J.S. (1962). A restatement of the zeroth law of thermodynamics, Am. J. Phys. 30: 294-296.
  10. ^ Truesdell, C.A. (1980). The Tragicomical History of Thermodynamics, 1822-1854, Springer, New York, ISBN 0-387-90403-4, page 262.
  11. ^ Maxwell, J.C. (1872). Theory of Heat, third edition, Longmans, Green, London, page 32.
  12. ^ Maxwell, J.C. (1872). Theory of Heat, third edition, Longmans, Green, London, page 186.
  13. ^ Tait, P.G. (1884). Heat, Macmillan, London, Chapters 21-22.
  14. ^ Tait, P.G. (1884). Heat, Macmillan, London, page 40.
  15. ^ Planck, M. (1897/1903). Treatise on Thermodynamics, translated by A. Ogg, Longmans, Green, London, page 2.
  16. ^ Fowler, R., Guggenheim, E.A. (1939/1965). Statistical Thermodynamics. A version of Statistical Mechanics for Students of Physics and Chemistry, Cambridge University Press, Cambridge UK.
  17. ^ Maxwell, J.C. (1872). Theory of Heat, third edition, Longmans, Green, London, pages 232-233.
  18. ^ Bailyn, M. (1994). A Survey of Thermodynamics, American Institute of Physics Press, New York, ISBN 0-88318-797-3, page 79.
  19. ^ Callen, G.B. (1960/1985). Thermodynamics and an Introduction to Thermostatistics, Wiley, New York.
  20. ^ Truesdell, C., Bharatha, S. (1977). The Concepts and Logic of Classical Thermodynamics as a Theory of Heat Engines, Rigorously Constructed upon the Foundation Laid by S. Carnot and F. Reech, Springer, New York, ISBN 0-387-07971-8.
  21. ^ Sommerfeld, A. (1951/1955). Thermodynamics and Statistical Mechanics, vol. 5 of Lectures on Theoretical Physics, edited by F. Bopp, J. Meixner, translated by J. Kestin, Academic Press, New York, page 1.
  22. ^ Guggenheim, E.A. (1949/1967). Thermodynamics. An Advanced Treatment for Chemists and Physicists and Chemists, fifth edition, North-Holland, Amsterdam, page 8.
  23. ^ Serrin, J. (1978). The concepts of thermodynamics, in Contemporary Developments in Continuum Mechanics and Partial Differential Equations. Proceedings of the International Symposium on Continuum Mechanics and Partial Differential Equations, Rio de Janiero, August 1977, edited by G.M. de La Penha, L.A.J. Medeiros, North-Holland, Amsterdam, ISBN 0-444-85166-6, pages 411-451.

Further reading